What is the max and min angles between the arms?

[dlang]

@bar, this should be easy to determine from the CAD, but hard to measure otherwise.

how far can the arms swing before they hit the vertical supports?

I realized that the repeated questions of ‘how close can the frame be to the workpiece’ aren’t just “things get worse as you get closer” but do have a hard limit. When the arms hit the vertical posts and are no longer in-line with the belt, the math for position can no longer be correct, so the max swing of the arms is a hard limit for frame vs workspace sizing (and it won’t be a constant distance, the wider the anchors are apart, the more distance you need)

[bar]

That is a great question. You are totally correct.

The minimum angle of the arms is 40 degrees and the maximum angle is 140 degrees.

[dlang]

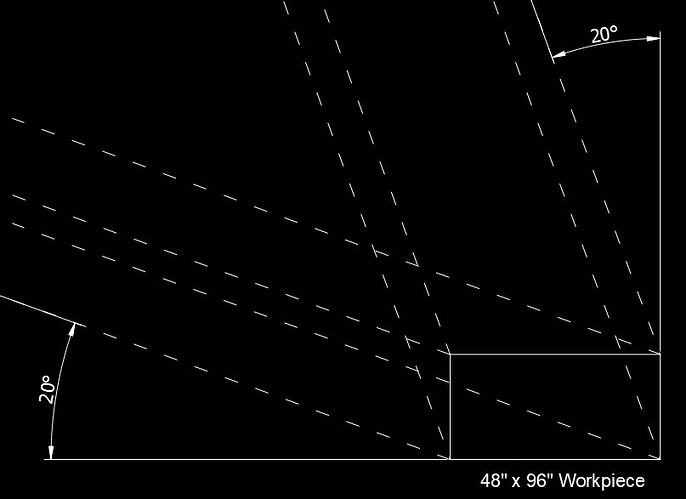
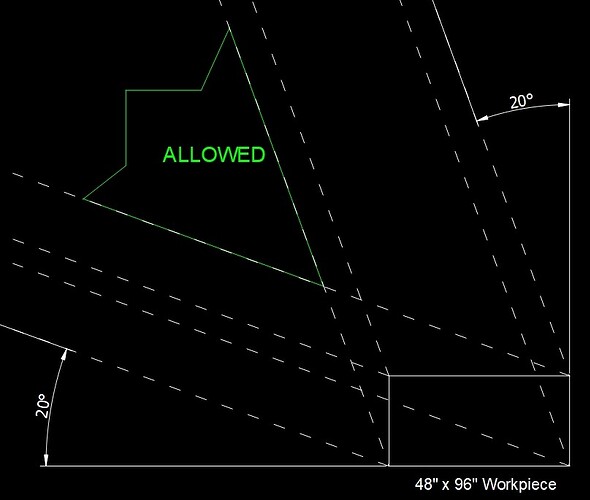
Ok, so 20 degrees from the vertical/horizonal plane. Since the verticals are
tapered, I assume this is at the widest part of the taper. Is this correct? If
so, the middle two arms would get just a little more movement than the top and
bottom anchors. If my math is correct, this difference could be significant.

Please check my math

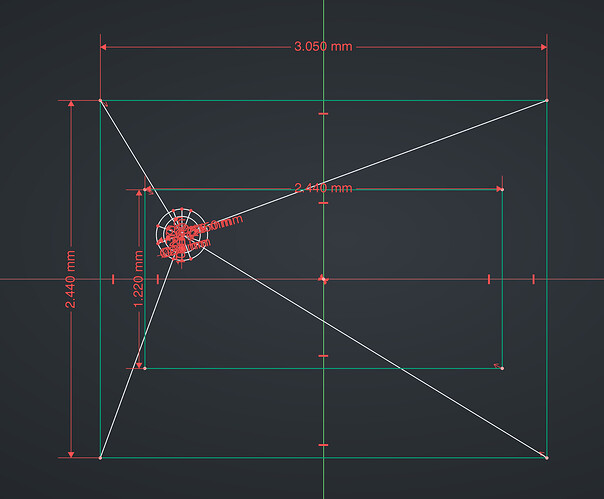
8x10’ frame

distance from top center to anchor 5’ horizontal 2’ vertical
ratio .4
inv tan(2/5) = 21.8 degrees works barely

distance from side center to anchor 1’ horizontal 4’ vertical
ratio .25
inv tan(1/4) = 14 degress, can’t actually work there

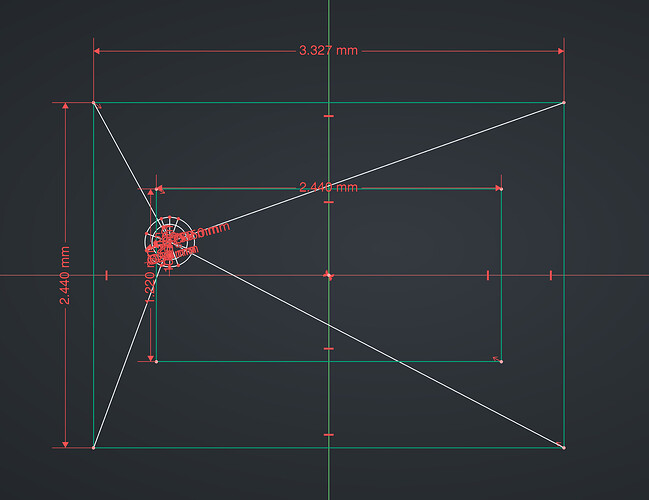
let’s try a 8x12 frame

top center 6’ 2’
ratio .33
inv tan(2/6) = 18 degrees, can’t actually work there

side center 2’ 4’
ratio .5
inv tan(2/4) = 26.5 degrees works

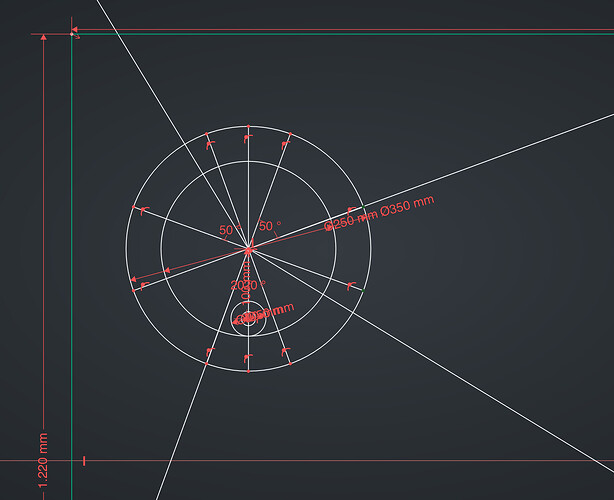
tan(20) = .364, so you need the ratio between the two numbers to be above this,
or to put it anothe way, if you have 2.75’ or more offset from the center of the
workpiece to the anchor for every 1’ in the other direction, you run into
problems

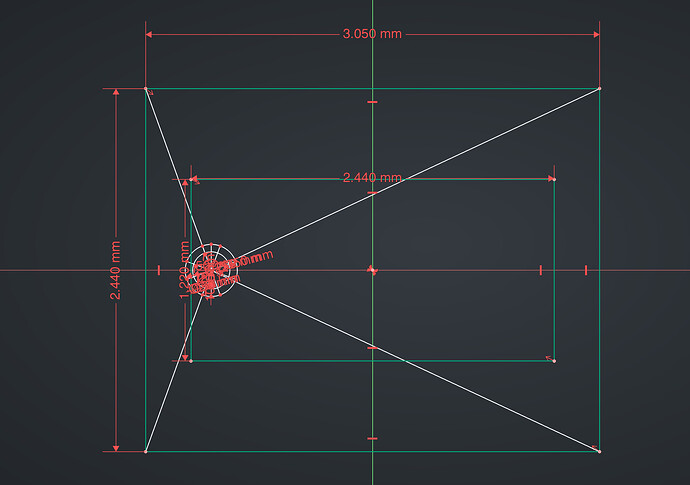
Setting up a quick spreadsheet and trying just a couple numbers, it looks like a
11’ x 8’ frame or 18" off the sides and 24" off the top and bottom results in
angles of 19.98 degrees and 20.56 degrees

This is close enough that differences between the CAD and the injection molds,
draft angle on the arms, and additional clearance provided by the taper in the
verticals will determine if it will work or just run into grief at the centers
of the edges.

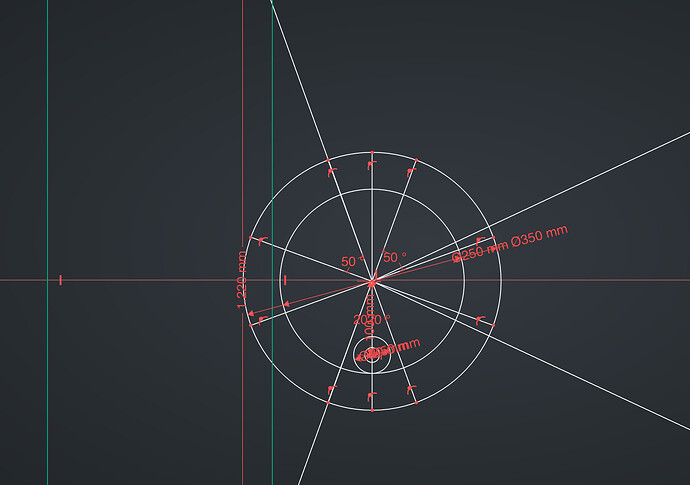
the shields around the lead screws can be made narrower (or eliminated??), to
allow the arms a little more movement (and since the sled can rotate, it would
not only increase how narrow the belt angles can get at the top/bottom, but also
help a little on how wide they can get on the sides).

David Lang

[dlang]

note that my musings about being able to narrow the angle at the top don’t help much because the motors hit the top bracket.

[arjenschoneveld]

hi Bar, is it really true that the min angle of two arms is 40 degrees? that would mean that the pivot angle of each arm is 50 degrees?
I drew that up, and that would mean that the minimum size of a frame to cut a 1220x2440 would have to be approximately 3800x4500. If the frame is smaller, some of the arms will collide with the uprights, in the corners of the workpiece.
For a frame of 3050x2440 this would mean that almost half of the work area will have issues with arms colliding with the uprights, and maybe this is what you are seeing in the callibrations.
I really hope that the pivot angle is more than the 50 degrees mentioned above, 60 degrees would be so much better: that would mean a frame of 2750x3660 would be good, slight issue in the far corners, but if the machine swivels a bit, no issue.
Could you measure the pivot angle of the arms again in your CAD?
Greetings,

Arjen

M4hoeken en framegrootte.FCStd (14.7 KB)

FreeCad!
just a sketch, you can move the sled aroundemphasized text

[bar]

I can double check, but I think that is right.

I think that it’s a concern, but it’s definitely not the source of the calibration issues that we’re tracking down. Those seem to happen even with a very small calibration pattern in the center of the sheet.

[WFD]

I was also wondering about the effect of the limits of the arm angles on the anchor locations. If I have a 4’ x 8’ workpiece and draw in the allowed limits on the angles of the upper left arm when the Maslow is at the four corners of the work piece I get this:

If I then show the allowed anchor location area for the top left anchor I get:

If I then draw in a 13’ radius representing a belt fully extended from the lower right corner (worst case) then I get:

It seems like it is not possible to have an anchor location that will allow you to access an entire 4’ x 8’ work piece without the arms interfering at some points.

Now I did not allow for the radius of the spool that the belt is wound on, so that might get you an anchor location, barely, or at least close, but then your frame dimensions would be almost 12’ high by 14’ wide.

Did I misunderstand something?

Sketched this in QCAD - file attached below.
20240404 Allowed Angles.dxf (32.0 KB)

[WFD]

And I should point out that a smaller work piece, like 4’ x 4’, would certainly work.

[WFD]

Maybe someone like @bar can test this by moving their Maslow to the four corners of a 4x8 and see if the arms interfere. In this example I think it woould be the upper right corner or the lower left.

[bar]

I can absolutely do that tomorrow. My experience is that at the extreme edges of the sheet they do touch, but not so much that its risen to the top of my list of concerns.

It’s more of an issue on a 10’ frame than a 12’ frame.

[dlang]

one thing you are missing is that the sled can rotate, so the issue isn’t the
absolute angle between the anchor and the sled, but rather the angle between the
two belts on each side.

that angle is at the max at the midpoint on the side. As you move from that
midpoint towards either anchor, the angle to the nearer anchor changes faster
than the angle to the far anchor.

re-do your work with this in mind and I think you will get different results.

David Lang

[dlang]

It’s mot an issue in the corners because the sled can rotate. But on a 8x10’
frame they will touch on the center of the sides and limit you, on a 8x12’ frame
they will touch on the center of the top/bottom and limit you.

a 8x11’ frame comes within a hair of working, a 8’x10’11" works.

David Lang

[dlang]

Also, there is the constraint that the belt must reach from the anchor to the
far corner (just over 13.5 ft)

David Lang

[arjenschoneveld]

you are right for the midpoint on the side, there the two arms on the same side will collide with the uprights. notice how the sled can’t reach the actual midpoint without the colliding of two arms with the uprights, rotating of the sled will not change this

but in the corner area its the opposit arms that collide with the uprights. notice how the sled can’t reach the corner without arms colliding with uprights:

With the 20 degree angle, the frame will have to be much bigger to have no collision issues, 3800x4500. edit: this is not correct, 2855 x 4492 is the lowest frame possible, if you go higher it can be less wide

Arjen

[arjenschoneveld]

On a 10’11" frame, it is slightly better: the midpoints of the sides are reachable without collisions, but the (large) corner areas are still a problem:

With some rotation of the sled, you are able to use an ellips shaped area of 4’ by 8’. The callibration grid should be placed within that area.

greets,

Arjen

[dlang]

are you saying that as you near the top left corner, the angle between the top
right and bottom left belts gets below 110 degrees? that I didn’t check

I’m not quite making out your diagrams, sorry.

David Lang

[arjenschoneveld]

it gets below 130 degrees (20 +90 +20)

[arjenschoneveld]

I am sorry that my diagrams are not clear enough, I will try to explain

I drew the sled , and in each quadrant of the sled the 50 degree swivel boundaries of each arm.
I drew the 4x8 work area, and the 10’11"x8 frame
I drew the belts from each frame corner to the center of the sled
I can move the sled over the workarea, and immediately see if the belts are in the 50 degree swivel areas, or in the 40 degree uprights areas

I hope this explains it better

Arjen

[dlang]

On a 10’11" frame, it is slightly better: the midpoints of the sides are reachable without collisions, but the (large) corner areas are still a problem:

hmm, it looks like if we cut off the top/bottom ears of the top clamp and
eliminate the shields around the lead screws, that would get let us get the arms
down to ~30 degrees from each other top and bottom

that should let us get the angle between opposite belts down to 105 degrees.

how close does that get us?

With some rotation of the sled, you are able to use an ellips shaped area of 4’ by 8’. The callibration grid should be placed within that area.

is it really an elipse? I am imagining it more like a 4 leaf clover, pinch
points at the middles where the two adjacent anchors hit the max angle, then it
expands before hitting the min angle near the top corners.

but I’ve been wrong in my visualizations before, which is why I was wanting to
graph it.

David Lang

[dlang]

right, I was thinking 90 + 20, forgetting that the dead zone is 40 degrees wide,
so it’s 90 + the dead zone.

how much closer can we get with the extra 5 degrees on the near vertical belt?

David Lang